An Inequality About Harmonic Mean Geometric Mean Arithmetic Mean

Arithmetic Mean Geometric Mean Harmonic Mean Youtube In mathematics, the qm am gm hm inequalities, also known as the mean inequality chain, state the relationship between the harmonic mean, geometric mean, arithmetic mean, and quadratic mean (also known as root mean square). suppose that are positive real numbers. then. these inequalities often appear in mathematical competitions and have. Visual proof that (x y)2 ≥ 4xy. taking square roots and dividing by two gives the am–gm inequality. [1] in mathematics, the inequality of arithmetic and geometric means, or more briefly the am–gm inequality, states that the arithmetic mean of a list of non negative real numbers is greater than or equal to the geometric mean of the same.

How To Prove Arithmetic Mean Geometric Mean Inequality Youtube The mean inequality chain, also called the rms am gm hm inequality, relates the root mean square, arithmetic mean, geometric mean, and harmonic mean of a list of nonnegative reals. in particular, it states that with equality if and only if . as with am gm, there also exists a weighted version of the mean inequality chain. power mean inequality. Here are some special cases of the power mean inequality: • p 1 ≥ p 0 (the am gm inequality). • p 0 ≥ p −1 (the gm hm inequality — hm is for “harmonic mean”). • p 1 ≥ p −1 (the am hm inequality). date: november 7, 1999. 1the reason for this convention is that when r is very small but nonzero the value of p r is very close to. The first derivative is f ′ (x) = ex − 1 − 1 and the second derivative is f ″ (x) = ex − 1. f is convex everywhere because f ″ (x)> 0, and has a minimum at x = 1. therefore x ≤ ex − 1 for all x, and the equation is only equal when x = 1. using this inequality we get. x1 a x2 a ⋯xn a ≤ ex1 a − 1ex2 a − 1⋯exn a − 1. Then, we can say that the limit as x goes to 0 is the geometric mean of the numbers. the quadratic mean's root mean power is 2 and the arithmetic mean's root mean power is 1, as and the harmonic mean's root mean power is 1 as . similarly, there is a root mean cube (or cubic mean), whose root mean power equals 3.

Prove The Inequality Of Arithmetic And Geometric Means Am Gm The first derivative is f ′ (x) = ex − 1 − 1 and the second derivative is f ″ (x) = ex − 1. f is convex everywhere because f ″ (x)> 0, and has a minimum at x = 1. therefore x ≤ ex − 1 for all x, and the equation is only equal when x = 1. using this inequality we get. x1 a x2 a ⋯xn a ≤ ex1 a − 1ex2 a − 1⋯exn a − 1. Then, we can say that the limit as x goes to 0 is the geometric mean of the numbers. the quadratic mean's root mean power is 2 and the arithmetic mean's root mean power is 1, as and the harmonic mean's root mean power is 1 as . similarly, there is a root mean cube (or cubic mean), whose root mean power equals 3. Two numbers. a geometric construction of the three pythagorean means of two numbers, a and b. the harmonic mean is denoted by h in purple, while the arithmetic mean is a in red and the geometric mean is g in blue. q denotes a fourth mean, the quadratic mean. Most recent test. however, there are other means besides the arithmetic mean. a less commonly known mean is the geometric mean. tonight we will investigate the geometric mean, derive the arithmetic mean geometric mean (am gm) inequality and do challenging problems. 2 review of the arithmetic mean and introduc tion to the geometric mean.
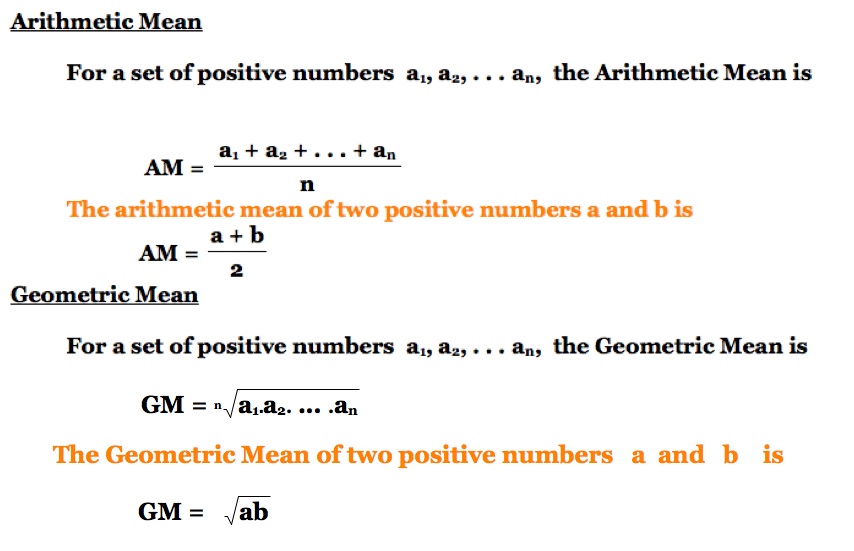
Using The Arithmetic Mean Geometric Mean Inequality In Problem Solving Two numbers. a geometric construction of the three pythagorean means of two numbers, a and b. the harmonic mean is denoted by h in purple, while the arithmetic mean is a in red and the geometric mean is g in blue. q denotes a fourth mean, the quadratic mean. Most recent test. however, there are other means besides the arithmetic mean. a less commonly known mean is the geometric mean. tonight we will investigate the geometric mean, derive the arithmetic mean geometric mean (am gm) inequality and do challenging problems. 2 review of the arithmetic mean and introduc tion to the geometric mean.

Comments are closed.